In this problem we find the eigenfunctions and eigenvalues of the differential equation $$\frac{d^2y}{dx^2} + \lambda y = 0$$ with boundary conditions $$y(0)+y^\prime(0) = 0 \hskip {20pt} y (3) = 0$$ For the general solution of the differential equation in the following cases use A and B for your constants, for example $y = A\cos (x) + B\sin(x)$. For the variable $\lambda$ type the word lambda, otherwise treat it as you would any other variable.

Case 1: $\lambda = 0$

(1a.) Ignoring the boundary conditions for a moment, the general solution of the differential equation is

Apply the boundary conditions to the general solution to obtain two equations relating $A$ to $B$:
(1b.) Solving this system for $A$ and $B$ we get
Case 2: $\lambda < 0$

(2a.) Ignoring the boundary conditions for a moment, the general solution is

Apply the boundary conditions to obtain two equations relating $A$ to $B$:

(2b.) Assuming $A$ and $B$ are not both zero then, solving these equations, one can eliminate both $A$ and $B$ and derive the following formula for $\sqrt{-\lambda}$ in terms of hyperbolic functions ($\cosh, \sinh, \tanh, \coth$, etc.).

(2c.) Choose the graph of $\sqrt{-\lambda}$ and $\tanh\left(3\sqrt{-\lambda}\right)$
A
B
C
D


From the graphs there are negative eigenvalues $\lambda$. They are

Sturm-Liouville guarantees infinitely many eigenvalues, so we go on.

Case 3: $\lambda > 0$

(3a.) Ignoring the boundary conditions for a moment, the general solution is

Apply the boundary conditions to get two equations relating $A$ to $B$:

(3b.) Assuming $A$ and $B$ are not both zero then, solving these equations, one can eliminate both $A$ and $B$ and derive the following formula for $\sqrt{\lambda}$ in terms of trigonometric functions ($\cos, \sin, \tan, \cot$, etc.).

In increasing order, the first four positive eigenvalues from this equation are: (Your answers should be accurate to at least 8 decimal places.)

(4.) List the first three eigenfunctions in order of increasing eigenvalues, including functions with negative eigenvalues.
$y_1(x) =$,
$y_2(x) =$,
$y_3(x) =$

Here are graphs of the first four eigenfunctions on $\lbrack 0,3 \rbrack$.

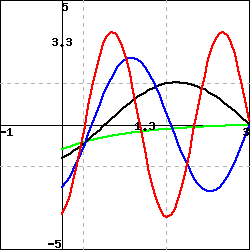
List the functions, ordered by their eigenvalues in increasing order, by their color - green, red, blue, black: