Suppose for and is a linear transformation.
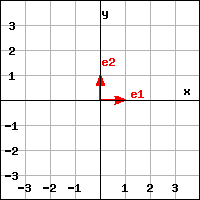 |
|
|
|
 |
|
|
Domain |
|
|
|
Codomain |
- Find a formula for . Your answer should be a coordinate vector, something of the form with functions in its components.
- Find the matrix for the linear transformation (relative to the standard
basis in the domain and codomain). That is, find the matrix such that . For instance, enter [ [1,2], [3,4] ] for the matrix .
- Find the kernel of . Enter your answer as a vector with constant entries, a vector with the variables or (or both) in its components (using a minimum number of variables), or enter R^2 for .
- Find the image of . Enter your answer as a vector with constant entries, a vector with the variables or (or both) in its components (using a minimum number of variables), or enter R^2 for .
- The linear transformation is (select all that apply):
You can earn partial credit on this problem.